Puzzle 1: Stare out a window and imagine that the objects you see are actually two dimensional shapes imbedded in the window glass. The window glass is thus a two dimensional world. Under what condition can, say, two car shapes move through each other without collision? Alternatively think of watching a movie. The image you see is really two dimensional. Nevertheless, if two cars are heading towards each other on the screen, you can tell whether they are going to crash or not. There is no true depth to the image, it's 2-D; what gives the illusion of depth? In other words, in this image what constitutes the third dimension?

.

Puzzle 2: it would seem that flatlanders cannot have a complete digestive system in the form of a tube running the length of their bodies for such a tube cuts them in half. Is there any way around this problem?

.

Puzzle 3: Abbott's Flatland is not really a very close analogue of our world, for although our space is 3-D we cannot move freely in three dimensions. Instead we must walk around on the surface of a sphere. What would be an analogous design for a 2-D world?

.

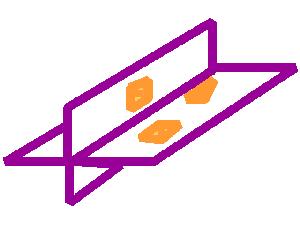Puzzle 4: In four dimensions it would be possible to have two 3-D spaces perpendicular to each other. Two such spaces would have only a plane in common. Suppose now there is a 3-D space perpendicular to ours, a space with people moving around in it. Use a flatland analogy to figure out how these people would appear to us. Alternatively, figure out how we would appear to them.

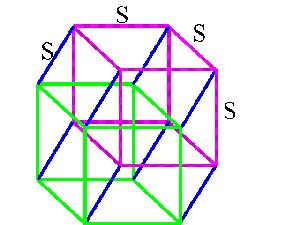Puzzle 5: The volume of a cube s feet on a side is s^3. What do you think the formula for the hypervolume of a hypercube s feet on a side should be. In particular, what is the hypervolume of a 2x2x2x2 hypercube?

All of these puzzles were adapted from Puzzles in Rucker's book. For more information about the fourth dimension or for more puzzles purchase this book or check it out at your library.