Here are the triangles Mod n for n=2, 3, 4, 5, 6, 7, 8, 9, 10, 11, and 12.

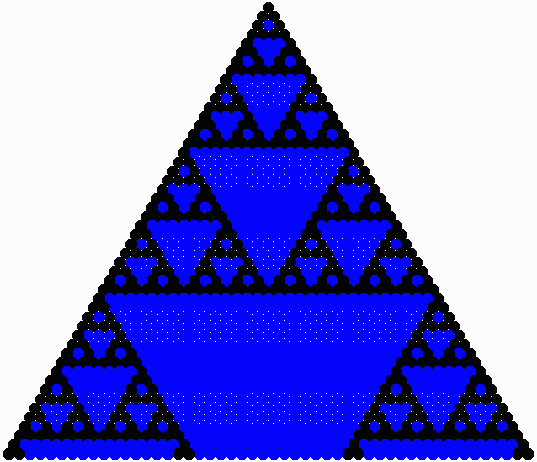
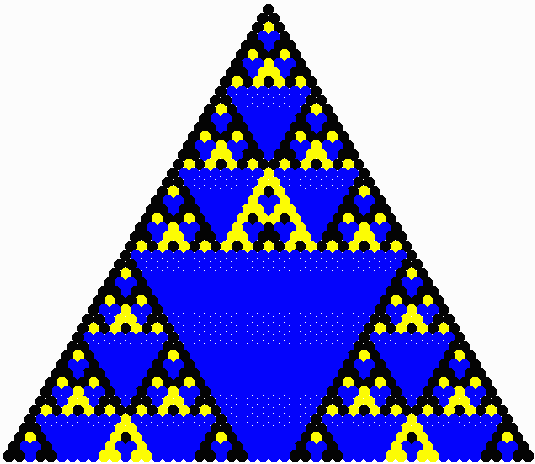
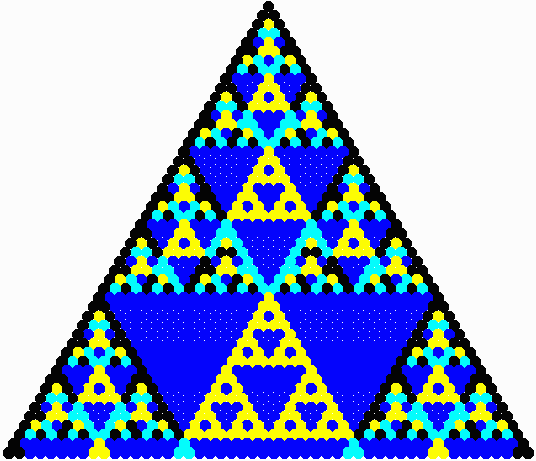
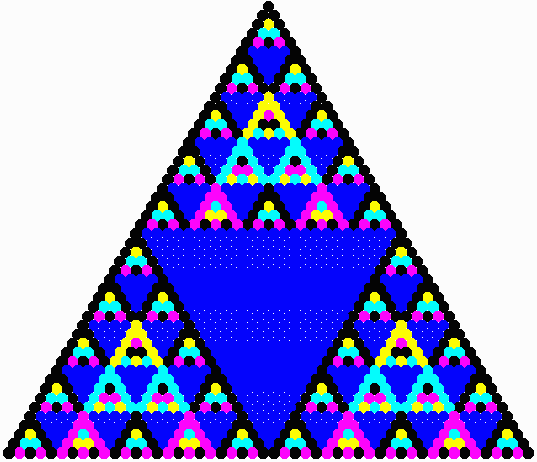
Pascal's triangle mod 2 with 50 rows Pascal's triangle mod 3 with 50 rows Pascal's triangle mod 4 with 50 rows Pascal's triangle mod 5 with 50 rows
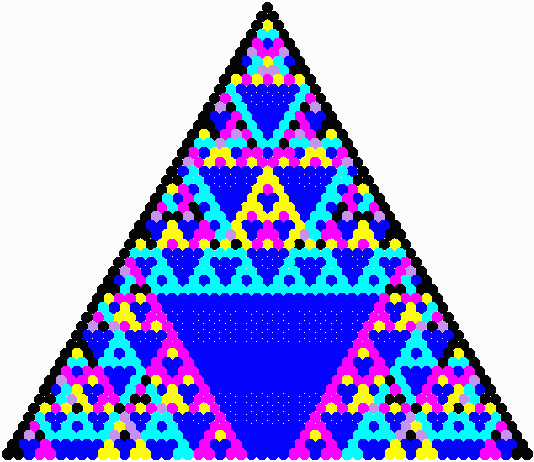
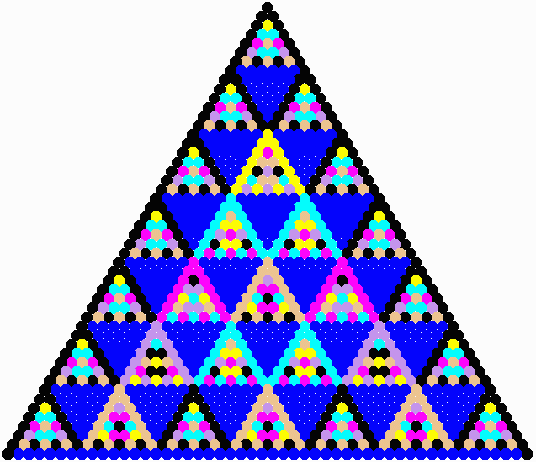
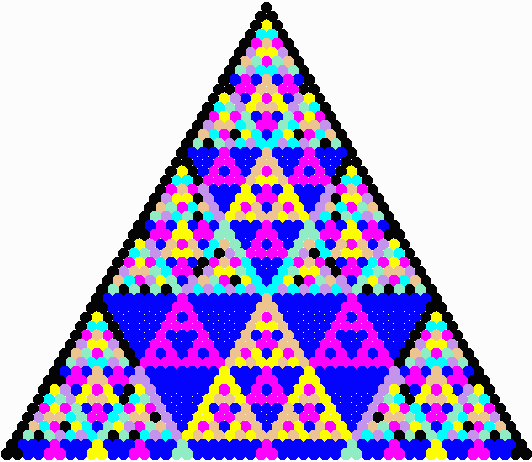
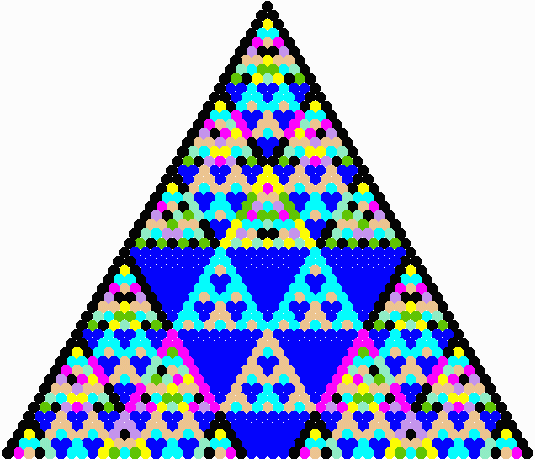
Pascal's triangle mod 2 with 50 rows Pascal's triangle mod 3 with 50 rows Pascal's triangle mod 4 with 50 rows Pascal's triangle mod 5 with 50 rows
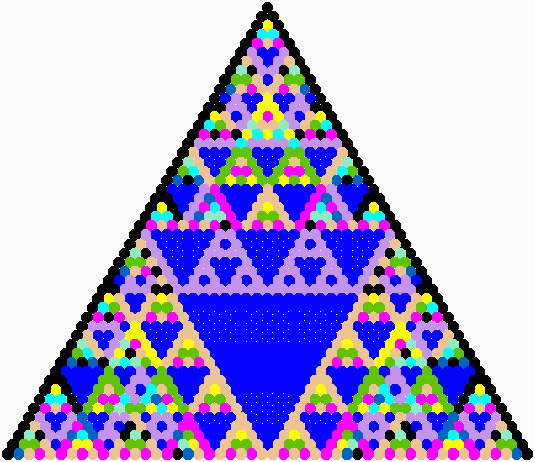
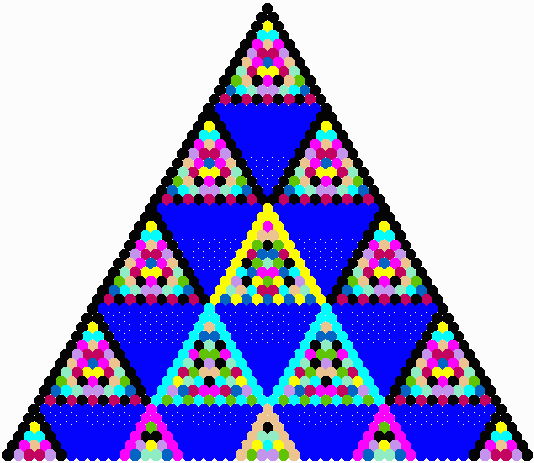
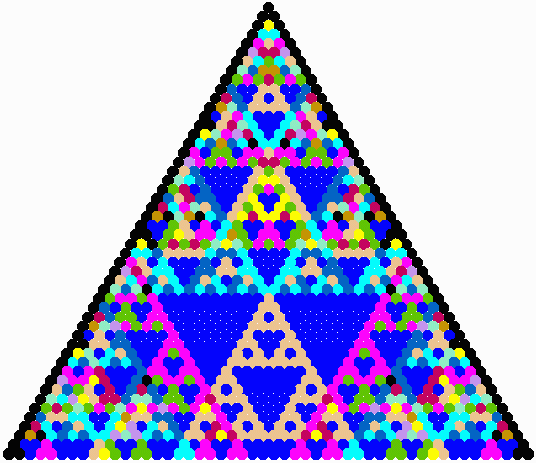
Pascal's triangle mod 2 with 50 rows Pascal's triangle mod 3 with 50 rows Pascal's triangle mod 4 with 50 rows colors assigned to 0 through 12
Look at the triangles for n=2 and n=4. See how similar they are? If you were to recolor the yellow circles as royal blue and the light blue circles as black, the n=4 triangle would be the same as the n=2 triangle. Why do you think that might be?
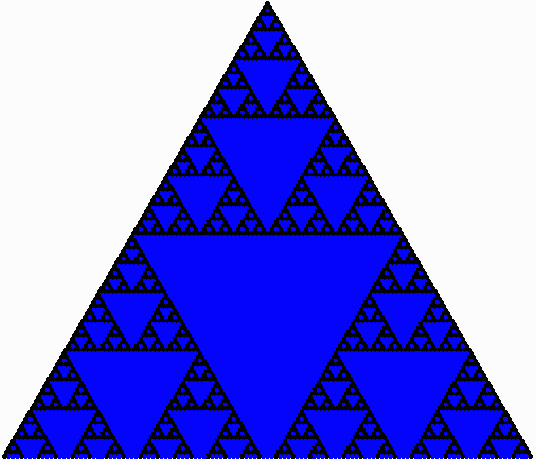
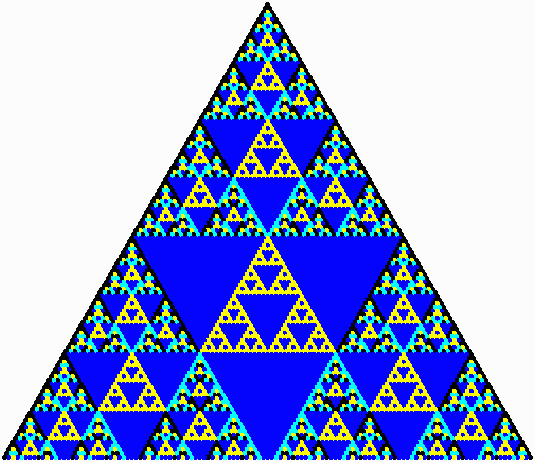
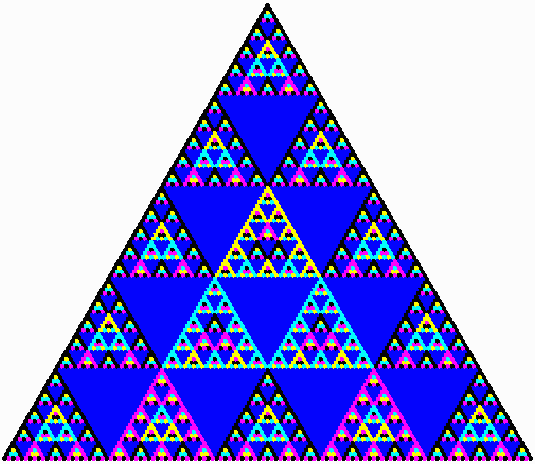
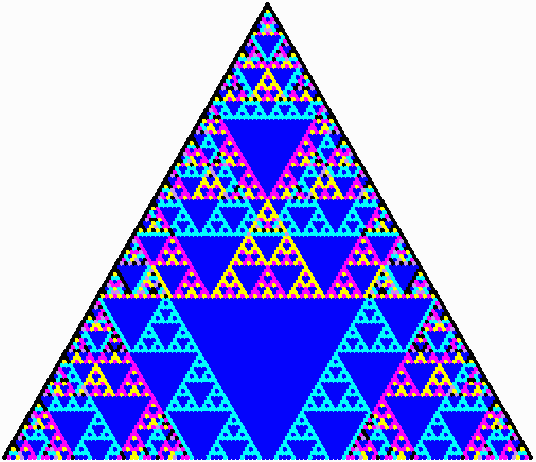
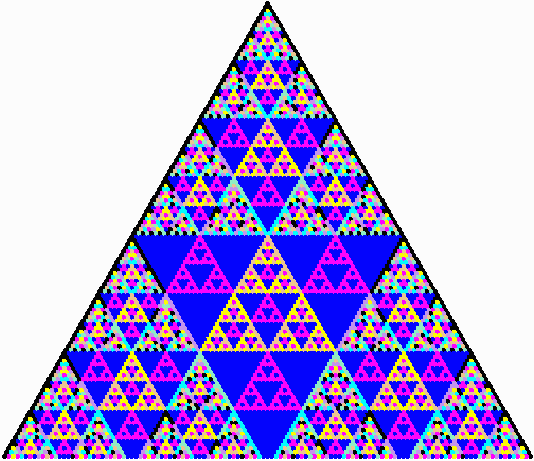
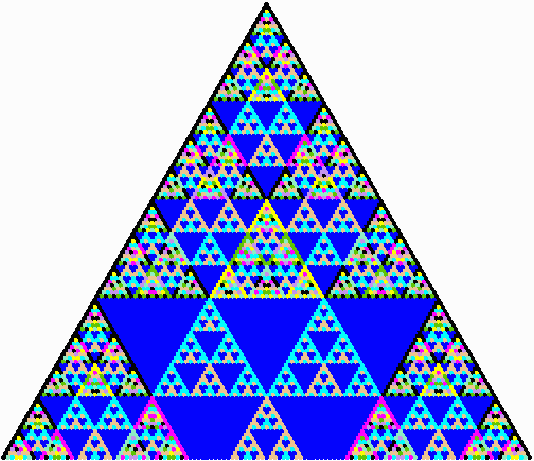
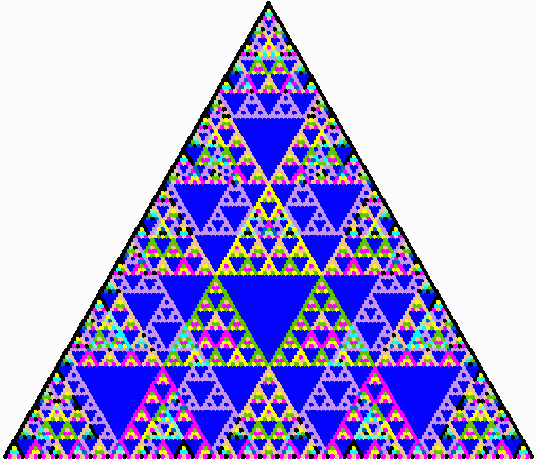
Faculty Notes Now look at the n=6 triangle. Can you see a color identification that would transform it to the n=2 triangle? How about to the n=3 triangle?

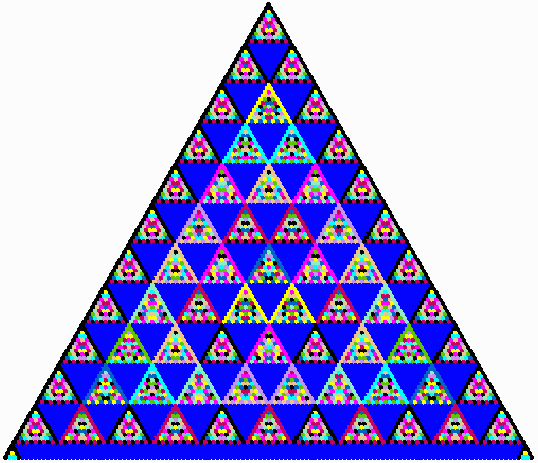
Now look at the n=8 triangle. Can you make an identification that would transform it to the n=4 triangle? You could then make another identification to transform it to the n=2 triangle.

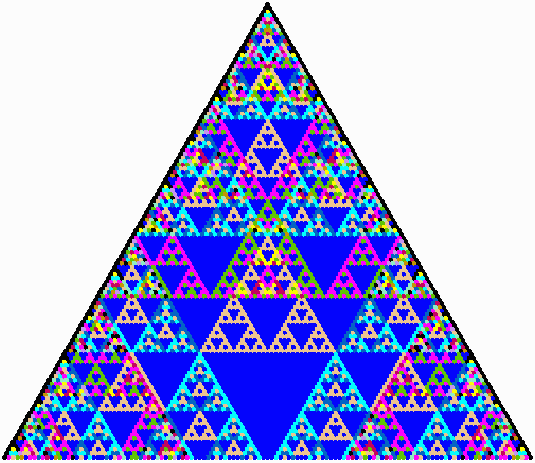
Can you see identifications between other triangles? What is the pattern here?

You might also like to see the triangles drawn to more rows:
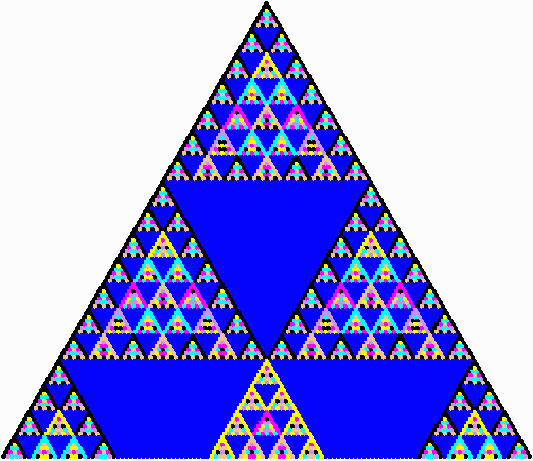
Pascal's triangle mod 2 with 125 rows Pascal's triangle mod 3 with 125 rows Pascal's triangle mod 4 with 125 rows Pascal's triangle mod 5 with 125 rows
Pascal's triangle mod 2 with 125 rows Pascal's triangle mod 3 with 125 rows Pascal's triangle mod 4 with 125 rows Pascal's triangle mod 5 with 125 rows
Pascal's triangle mod 2 with 125 rows Pascal's triangle mod 3 with 125 rows Pascal's triangle mod 4 with 125 rows colors assigned to 0 through 12

One of the aspects of the patterns that is immediately noticeable is the existence of downward pointing royal blue triangles. Since in all of these triangles royal blue corresponds to the number zero, you can see that whenever you get a string of zeros on a row this will generate a downward pointing blue triangle. Any entry below two zeros will be zero. But the non-zero entries on either end of the string will encroach one position on each side for each row. Try it: start with a row {1,0,0,0,0,0,1} and generate rows below using the Pascal's triangle rule. You get:

1

 

0

 

0

 

0

 

0

 

0

 

1

 

1

 

0

 

0

 

0

 

0

 

1

 

 

 

1

 

0

 

0

 

0

 

1

 

 

 

 

 

1

 

0

 

0

 

1

 

 

 

 

 

 

 

1

 

0

 

1

 

 

 

 

 

 

 

 

 

1

 

1

 

 

 

 

 

 

 

 

 

 

 

2

 

 

 

 

 

 
The downward pointing triangles containing subsets of the colors for a triangle occur for similar reasons. For example, look at one of the blue triangles for the mod 2 triangle. Now look at the same area in the mod 4 and mod 8 triangles. What do you see? Look at the same region in the mod 6 triangle. What can you say about the colors in this region in the mod n triangles for any even mod?