MATH 230 Fundamental Concepts II
Spring 2007 Assignments and Tests

 

View the "Guidelines for Written Work."

Each assignment you submit must be enclosed in a cover sheet employing a prescribed format. Information on "The Prescribed Format for Assignment Cover Sheets" can be found by following one of the following links. Submit written solutions for the exercises in parentheses and any other exercises where you are instructed to provide a written solution.  You should do the other exercises for practice or self-testing.  All assignments are due at the beginning of the class period on the assigned due date.  No late papers will be accepted.

 

The Prescribed Format for Assignment Cover Sheets (Internet Explorer)

 

Go directly to:

Return to the homepage for this course .
Look at the " Guidelines for Written Work ."

 

Assignment #1 - Due on Thursday, Feb. 8

 

1.)  If you have not already done so, activate your computer account.

 

2.)  Log in on the campus computer system and do the following:

a) Read any incoming e-mail from your instructor you find in your mail box.
b) Send an e-mail message to your instructor.  If you choose to use an e-mail account other than your SU GroupWise account, you may do so.  However, you must arrange to have your e-mail to your campus account forwarded to your preferred account. "Speedy Sheets" with instructions for using GroupWise can be found at http://helpdesk.salisbury.edu/informationCenter/documentation/speedysheets/speedysheets.htm#GroupWise.


3.)  Access the home page for this course.  (http://faculty.salisbury.edu/~dccathcart/Math230/Math230Home.html)


4.)  Follow the appropriate links and read the letter "To the Student, the "Course Syllabus," your "Instructor's Policies," and the "Guidelines for Written Work."

 

5.)  Read the indicated sections in your text and work the exercises indicated below.  Turn in written solutions for only the exercises indicated in parentheses.   When you submit your paper, be sure it is enclosed in a cover sheet employing the prescribed format. Information on "The Prescribed Format for Assignment Cover Sheets" can be found by following one of the links on the assignment page for this course.. Submit written solutions for the exercises in parentheses and any other exercises where you are instructed to provide a written solution.  You should do the other exercises for practice or self-testing.  All assignments are due at the beginning of the class period on the assigned due date.  No late papers will be accepted.

 

Read, and work through, Sections 6.1-6.3 in the text.  It is assumed that you already have mastered the skills and concepts addressed in those sections.  So, most of that material will not be presented in class.  It will be your responsibility to ask questions in class about any material in those sections that you find confusing.  Turn in written solutions only for the "regular exercises" and "problem solving exercises" whose numbers are enclosed in parentheses.  Write up your regular exercise solutions in a step-by-step manner so your thought process is clear.  (Regular exercises are not designated by the "PS" icon.)  Write up your problem solving exercise solutions employing Polya's four-step process demonstrated in the problem problem solutions on p. 381 and on p. 405-6.)  Problem solving exercises are designated by the "PS" icon.)

 

Exercises and Problems 6.1:  3-39 odd, (38)

Exercises and Problems 6.2:  (4), 11-25 odd, (28), 29

Exercises and Problems 6.3: 1-23 odd, (34), 35, (36), 37, (38)

Look at a sample write up for 6.2 #4

Look at another sample write up for 6.2 #4

Look at a sample write up for 6.3 #34

Look at a sample write up for 6.3 #36

Look at a sample write up for 6.3 #38

 

Look at the " Guidelines for Written Work ."
Look at Polya's Problem Solving Process .
Return to the top of this page .
Look at the homepage for this course .

 

Assignment #2 - Due on Thursday, Feb. 15

 

Turn in written solutions only for the "regular exercises" and "problem solving exercises" whose numbers are enclosed in parentheses.  Write up your regular exercise solutions in a step-by-step manner as illustrated in the sample solutions for Examples K and L on page 387 in the text.  (Regular exercises are not designated by the "PS" icon.)  Write up your problem solving exercise solutions employing Polya's four-step process demonstrated in the problem problem solution on pp. 394-395.)Problem solving exercises are designated by the "PS" icon.)

 

Read and work through Section 6.4 in "Mathematics for Elementary Teachers:  A Conceptual Approach."

Exercises and Problems 6.4:  1-21 all the odds, (14), (16), 33, (38), (42) For #42 you will graph the function defined by f(x) = √x in the manner illustrated in Section 2.2 of your textbook. 

Chapter 6 Test (pp. 422-423):  3, 7, 8, 9, 10, 11, 12, 13, 15, 16, 18, (20) 

Look at a sample write up for 6.4 #38

Look at a sample write up for 6.4 #42

Look at a sample write up for CH 2 Test #20

 

Assignment #3 - Due on Thursday, Feb. 22

 

Turn in written solutions only for the exercises whose numbers are enclosed in parentheses. Write up your problem solutions for "PS" exercises in a step-by-step manner employing Polya's four-step process.

Exercises and Problems 9.1:  3-21 odd, (4), 22, 23, (24), 25, (28) Hint for #28: Look at #22 and #23.

Read the indicated sections and work the indicated exercises. 

Read and work through Section 9.2  in the text.
Exercises and Problems 9.2:  3-19 odd, (4), (6), 7, (12), (18), (26)

Look at a sample write up for 9.1 #24

Look at a sample write up for 9.1 #28

Look at another sample write up for 9.2 #4

Look at a sample write up for 9.2 #18

Look at a sample write up for 9.2 #26

Look at example of an excellent paper.

 

Return to the top of this page .

Assignment #4 - Due on Thursday, March 1

Exercises and Problems 9.2:  (14, Justify your answers.), (28)

Look at a sample write up for 9.2 #28

Look at a student's write up for this assignment

Study Guide for Test #1 - Thursday, March 1

Review the "MATH 230-001 Course Objectives."

The test will cover objectives 6.1.1 through 6.4.5 and 6.* inclusive and objectives 9.1.1 through 9.2.1 and 9.* inclusive.  You should work and check as many of the suggested exercises as possible, and come to class sessions prepared to ask questions about those exercises or objectives you do not understand.  You may also e-mail questions to your instructor.

No questions concerning Test #1 will be answered after Tuesday, Feb. 27.   No protractor, ruler, compass, or calculator can be used on the test.  (You may use your polygon pieces.)

Link to a copy of Test #1 given in Fall '06.  No solutions will be given for items on this sample test.

Look at Test #1, Fall '06 with sample solutions.

 

Assignment #5 - Due on Tuesday, March 6

 

Do the following:

Construct models of five polyhedra using the patterns on Material Cards 29 and 30 in your spiral-bound activity book.  You may need to look at pictures of those polyhedra to see how the models should be assembled.  Bring those models to class on Tuesday,  March 6.  Also, bring your spiral-bound activity book. 

 

Assignment #6 - Due on Thursday, March 8

 

Turn in written solutions only for the "regular exercises" and "problem solving exercises" whose numbers are enclosed in parentheses.

Exercises and Problems 9.3:  2-13 all, 27, (28) (Instructions for #28 are on page 618 just above exercise #27.), 29, (30), (34)

Bring your spiral bound activity book and your pattern block pieces to class on Thursday, March 8. 

Look at some sample write-ups for this assignment.

 

Assignment #7 - Due on Thursday, March 15

 

Work through Section 9.4 in your textbook.

Exercises and Problems 9.4:  (2), 3-17 odd, (6), (10), (14), (18a), (30)

Work through Section 10.1 in your textbook

Exercises and Problems 10.1:  1-5 odd, 6, 8-15 all, (14), (20), (32)

Look at a sample solution for 9.4/#10 & #14.

Look at a sample solution for 9.4/#18 & #30.

Look at a sample solutions for 10.1/#14 & #20.

Look at a sample solution for 10.1/#32.

Look at another sample solution for 10.1/#32.

 

Assignment #8 - Due on Thursday, March 29 or Tuesday, April 3  (Your choice.)

 

Read and work through Section 10.2 in the text.

Do Example B on page 671.

(Work #6 in the enrichment activity on page 684.)   

Exercises and Problems 10.2:  (6), 7a (Check your answer in the back of the book.), (8), (14), 13-21 odd, (18), (22), (26), (40)

Read and work through Section 10.3 in the text.

Exercises and Problems 10.3:  5-13 odd, (12  See instructions above #7.), (14  See instructions above #13.), (32) For #32 take a look at #28.

Activity Set 10.3 in Spiral-Bound Activity Book:  (5)

 

Look at a sample solution for 10.2/#22.

Look at a sample solution for 10.2/#26.

Look at a sample solution for 10.2/#40.

Look at a sample solutions for 10.3/#12 & #14.

 

Test #2 - Tuesday, April 3

Test #2 will address objectives for Chapters 9 and 10.  That is objectives 9.1.1-10.* in the list of course objectives at http://faculty.salisbury.edu/~dccathcart/Math230/objectiv04.html  (Calculators will not be allowed.)

A good way to prepare is to work many of the exercises suggested with the objectives.  As you work each assignment, work the suggested odd numbered exercises and check your answers by looking in the back of the text.  Many test items will look like those exercises assigned but not collected.  Problem solving ability, persistence, and creativity are evaluated on the papers you turn in each week.  The more routine knowledge, skill, and concept-related objectives are evaluated on the tests.

You will need to have formulas for perimeter, area, and volume memorized. (Calculators will not be allowed.)

 

Look at a copy of Test #2 given Spring '06. 

Link to solutions for some perimeter, area, and volume problems.

Look at Test #2, Spring '06 with sample solutions.

 

 

 

Assignment #9 - Due on Thursday, April 12

Look at the different problem solving strategies illustrated in Section 1.1.

Carefully study the sample problem that is stated, "Find an easy method for computing the sum of consecutive whole numbers from 1 to any given number.

Exercises and Problems 1.1:  (2) Look at exercise #1; (8) look at #5

Please remember to submit an example of your best work.  Papers are expected to be neat, well-organized, and correct.  Clearly communicate your thought processes and state your conclusions using complete sentences.

 

Assignment #10 - Due on Tuesday, April 17

Read and work through Section 1.2.

Exercises and Problems 1.2:  3, 5, (6 a PS exercise) Look at #5, 7, (8 a PS exercise) Look at #7, (24)

 

Look at a sample solution for 1.2/#6.

Look at a sample solution for 1.2/#8.

 

Assignment #11 - Due on Tuesday, April 24

 

Carefully work through Section 1.3 (pp. 36-46 in your textbook.)

Ex/Prob Set 1.3:  9, (10), 11, (36)  (Be certain you can solve equations like those in #9 and #11.)

Carefully work through Section 2.2.  (pp. 75-91 in your textbook.)

Carefully study Examples E, F, and G on pages 84-88 in your text.  Be sure you understand  how the

solutions were obtained. 

Ex/Prob Set 2.2:  (4), 9, 11, (12), 17, (24), (26), (38)

(Write up the following exercise:)

(Exercise)  Consider the following three sequences.  One is an arithmetic sequence; one is geometric, and

one is neither arithmetic nor geometric.  Draw a very nice graph of each sequence and then classify each

sequence by type, identify F(8) and show how to determine both a difference equation and an explicit functional

equation for each sequence.  Don't worry if you can't find an explicit function for (a).

(a)  

n 0 1 2 3 4 5 6 7
F(n) 5 10 19 32 49 70 95 124

 

(b)

n 0 1 2 3 4 5 6 7
F(n) 3 6 12 24 48 96 192 384

 

(c)

n 0 1 2 3 4 5 6 7
F(n) 4.00 5.50 7.00 8.50 10.00 11.50 13.00 14.50

 

Look at a sample solution for 1.3/#36.

Look at sample solutions for 2.2/#4&#12.

Look at sample solutions (except for the graphs) for the above exercises (a), (b), & (c).

 

Assignment #12 - Due on Tuesday, May 1

 

Carefully study the material on "Scatter Plots" on pp. 437-438 in your text.

In particular, study Examples E and F.

Ex/Prob Set 7.1:  (36), (38)  Be sure to display your graphs and write out the equations of your trend lines.

 

Look at a student's write up for this assignment

 

Study Guide for Test #3 - Thursday, May 3

Test three will address objectives 1.2.1, 2.2.1, 2.2.2, 2.2.3, M.1-M.6  (Calculators are allowed.) You may find the course objectives at

 http://faculty.salisbury.edu/~dccathcart/Math230/objectiv04.html  

 

A good way to prepare for a test is to work many of the exercises suggested with the objectives.  Also, as you work each assignment, work the suggested odd numbered exercises and check your answers by looking in the back of the text.  Many test items will look like those exercises assigned but not collected.  Problem solving ability, persistence, and creativity are evaluated on the papers you turn in each week.  The more routine knowledge, skill, and concept-related objectives are evaluated on the tests.


Link to a sample test - Test #3, Spring 2004.  Note that solutions will not be provided for items on this sample test.  Compare your solutions with those of another individual.  Keep studying until you are confident in your solutions. You may use a calculator on Test #3.

In addition to working the practice test, you need to be sure you can work problems like those on the assignments since Test #2 and like those we have worked in class since Test #2.  Link to another sample test - Test #3, Fall 2006.

Look at Test #3, Spring '06 with sample solutions.

Look at a student's work on a version of test #3 given in a past semester.

 

Assignment #13 - Due on Thursday, May 10

(Exercise 1.)  (Write up this exercise.)  These days it is possible to lease just about any car. For example, for the modest fee of $1500 down plus $750 per month you can drive a Jaguar. Unfortunately, those charges add up.

a) What pattern will appear in a table showing total leasing cost as a function of number of months in the lease? 

Lease time    0   1   2   3   4   5   6   7   8
Total cost
 
b) What pattern do you expect in a graph relating lease time and total payment?
c) What equation will give an appropriate rule relating length of lease (x) and total payment (y)?
 

(Exercise 2.)  (Write up this exercise.) In 1975 a new Ford Mustang car had a base price of $4,906. In 1981 a comparable car had a base price of $7,900; in 1987 a base price of $9,750; and in 1993 a base price of $13,245.
(a). On graph paper, make a plot of the sample (year, price) data and draw a line that fits the pattern of the data well. (Hint: Use time beginning with 1975 as 0.) Find an equation in the form y = mx + b for that model line and explain what m and b tell about the situation.
(b). Use your linear model from (a) to estimate base price for Ford Mustangs in 1978, 1984, 1990, and 1994.

Be sure to do you beast work in writing up theses two exercises.  Identify any variables you introduce and carefully state your conclusions.  These exercises are taken from a text written by Dr. James Fey at the University of Maryland.  (See http://www.towson.edu/csme/mctp/Courses/Mathematics/UnitIIIB.html)

 (Exercise 3.)  (Write up this exercise.)  Most popular American sports involve balls of some sort. In playing with those balls one of the most important factors is the bounciness or elasticity of the ball. For example, if a new golf ball is dropped onto a hard surface, it should rebound to about 80% of its drop height. Suppose a high shot drops downward from a height of 25 feet onto a road and keeps bouncing up and down again and again.



(a) Make a table and plot of the data showing expected heights of the first ten bounces. 

Bounce Number    0     1       2       3       4       5          6     7 ...    
Rebound          25    ...                                                       
Height  
                                                                        

(b) How does the rebound height change from one bounce to the next? How is that pattern shown by the shape of the data plot?

(c) Write a difference equation showing how to calculate the rebound height for any bounce from the height of the preceding bounce.

(d) Write a functional equation to estimate the rebound height after any number of bounces.

(e) How will the data table, plot, and equations for calculating rebound height change if the ball drops first from only 16 feet?

As is the case with all mathematical models, data from actual tests of golf ball bouncing will not match exactly the predictions from equations of ideal bounces. You can simulate the kind of quality control testing that factories do by running some experiments in your classroom.

(Exercise 4. )  (Write up this exercise.)  One of the amazing properties of mathematical ideas is that patterns discovered in one context turn up in quite different and unexpected places. Then calculations done the first time can be applied to the new situations. This recurrence of fundamental patterns is an especially impressive property of exponential models.

For example, drugs are a very important part of the human health equation. Many drugs are essential in preventing and curing serious physical and mental illnesses; many other drugs cause damaging addiction and physical or mental impairment.



For the 5 million Americans of all ages who suffer from diabetes, insulin is an extremely valuable drug. To provide that essential hormone, when the body's pancreas production fails, diabetics take the drug insulin. However, once in the blood stream, insulin begins breaking down into other chemicals and soon passes from the body. A typical pattern of decrease for insulin in the blood is shown in the following graph.



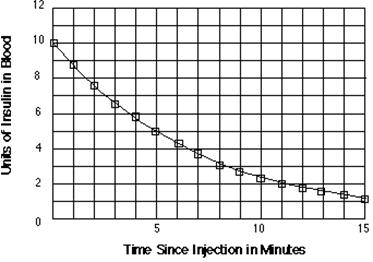
(a)  Medical scientists are usually interested in the time is takes for a drug to be reduced to one half of the original dose. They call this time the half-life of the drug. What appears to be the half-life of insulin?

The pattern of decay shown on the graph for insulin can be modeled well by an exponential equation in the form

y = A(b)x .

(b)  Enter five or six data points from the given graph and calculate the ratios of successive terms to the preceding terms.  Choose a particular ratio to represent the “typical” pattern of change you observe, and use that ratio to formulate both a difference equation and a functional equation to approximate the relationship between the amount of insulin in the blood and time elapsed since injection.

(c) Test your rules and describe how well your models fit the data in the graph.

(d) Explain what the values of A and b tell about the action of insulin in the blood.


 Look at a student's write up for this assignment

 

Final Exam - (Fri., May 18 at 8:00 am for Section 001; Tues, May 22 at 8:00 am for Section 002)

The final exam is comprehensive and will address the course objectives.  You may use a calculator on the final exam.
Review Test #1, Test #2, and Test #3 as you prepare for the final exam.  Be sure you can work items like those on
each of the three tests.

Link to a grade calculation worksheet.

Look at the " Guidelines for Written Work ."
Return to the top of this page .
Look at the homepage for this course .